| ds2 | = | 1-2M/r |
dr2 | + | r2d 2 - (1-2M/r)dt2, 2 - (1-2M/r)dt2, |
| Table of Contents | Back to Lecture 14: The Einstein Field Equations and Derivation of Newton's Law | On to Lecture 16: White Dwarfs, Neutron Stars, and Black Holes, by Gregory C. Levine |
15. The Schwarzschild Metric and Event Horizons
We saw that the metric outside a spherically symmetric static stable star (Schwarzschild metric) is given by
| ds2 | = | 1-2M/r |
dr2 | + | r2d 2 - (1-2M/r)dt2, 2 - (1-2M/r)dt2, |
where d 2 = d
2 = d 2 + sin2
2 + sin2 d
d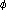 2. We see immediately that something strange happens when 2M = r, and we look at two cases.
2. We see immediately that something strange happens when 2M = r, and we look at two cases.
Case 1 (Not-So-Dense Stars) Radius of the star, rs > 2M.
If we recall that the Schwarzschild metric is only valid for outside a star; that is, r > rs, we find that r > 2M as well, and so 1-2M/r is positive, and never zero. (If r  2M, we are inside the star, and the Schwarzschild metric no longer applies.)
2M, we are inside the star, and the Schwarzschild metric no longer applies.)

Case 2 (Extremely Dense Stars) Radius of the star, rs < 2M. Here, two things happen: First, as a consequence of the equations of motion, it can be shown that in fact the pressure inside the star is unable to hold up against the gravitational forces, and the star collapses (see the next section) overwhelming even the quantum mechanical forces. In fact, it collapses to a singularity, a point with infinite density and no physical dimension, a black hole. For such objects, we have two distinct regions, defined by r > 2M and r < 2M, separated by the event horizon, r = 2M, where the metric goes infinite.

Particles Falling Inwards Suppose a particle is falling radially inwards. Let us see how long, on the particle's clock (proper time), it takes to reach the event horizon. Out approach will be as follows:

 to see how long it takes.
to see how long it takes.Recall first the geodesic equation for such a particle,
 risPrPs = 0.
risPrPs = 0.
We saw in the derivation (look back) that it came from the equation
| m0 | d  |
+ |  risPrPs = 0 risPrPs = 0 |
There is a covariant version of this:
| m0 | d  |
- |  risPrPi = 0. risPrPi = 0. |
Press here for a derivation.
Now take this covariant version and write out the Christoffel symbols:
| m0 | d  |
= |
|
||
| m0 | d  |
= |
|
||
| m0 | d  |
= |
|
But the sum of the second and third terms in parentheses is skew-symmetric in r and k, whereas the term outside is symmetric in them. This results in them canceling when we sum over repeated indices. Thus, we are left with
| m0 | d  |
= | 1 2 |
grk,sPrPk. |
But by spherical symmetry, g is independent of xi if i = 2, 3, 4. Therefore grk,s = 0 unless s = 1. This means that P2, P3 and P4 are constant along the trajectory. Since P4 is constant, we define
another constant.
Question What is the meaning of E?
b>Answer Recall that the fourth coordinate of four momentum is the energy. Suppose the particle starts at rest at r = ì and then falls inward. Since space is flat there, and the particle is at rest, we have
(which corresponds to P* = [0, 0, 0, -m0], since P* = P*g**). Thus, E = -P4/m0 = 1, the rest energy per unit mass.
As the particle moves radially inwards, P2 = P3 = 0. What about P1? Now we know the first coordinate of the contravariant momentum is given by
| P1 | = | m0 | d  |
(by definition, Pi = m0 dxi/d , and x1 = r) , and x1 = r) |
Thus, using the metric to get the fourth contravariant coordinate,
| P* | = | ( m0 | d  |
, 0, 0, m0E(1-2M/r)-1) |
we now invoke the normalization condition  u, u
u, u  = -1, whence
= -1, whence  P, P
P, P  = -m02, so that
= -m02, so that
| -m02 | = | m02 | d  |
2 |
(1 - 2M/r)-1 - m02E2(1-2M/r)-1, |
giving
d  |
2 |
= | E2 - 1 + 2M/r, |
which is the next step in our quest:
d = - = - |
(E2-1+2M/r)1/2 |
, |
where we have introduced the negative sign since r is a decreasing function of  . Therefore, the total time elapsed is
. Therefore, the total time elapsed is
| T | = | 2M R |
- | (E2-1+2M/r)1/2 |
, |
Now let's recalculate this from the point of view of an observer who is stationary with respect to the star. That is, let us use the coordinate x4 as time t. How is it related to proper time? Well, the four velocity tells how:
| V4 | =defn | d  |
= | d  |
We can get V4 from the formula for P* (and divide by m0) so that
 = E(1-2M/r)-1 d
= E(1-2M/r)-1 d
giving a total time of
| T | = | 2M R |
- | (1-2M/r) (E2-1+2M/r)1/2 |
, |
This integral diverges! So, in the eyes of an outside observer, it takes that particle infinitely long to get there!
Inside the Event Horizon -- A Dialogue
Tortoise:
 2 - (1-2M/r)dt2.
2 - (1-2M/r)dt2.
Achilles: Indeed, but notice that now the coefficient of dr2 is negative, while that of dt2 is positive. What could that signify (if anything)?
Tortoise: Let us do a little thought experiment. If we are unfortunate(?) enough to be there watching a particle follow either a null or timelike world line, then, with respect to any parameter (such as  ) we must have dr/d
) we must have dr/d
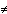 0. In other words, r must always change with the parameter!
0. In other words, r must always change with the parameter!
Achilles: So you mean nothing can sit still. Why so?
Tortoise: Simple. First: for any world line, the vector dxi/d is non-zero, (or else it would not be a path at all!) so some coordinate must be non-zero. But now if we calculate ||dxi/d
is non-zero, (or else it would not be a path at all!) so some coordinate must be non-zero. But now if we calculate ||dxi/d ||2 using the signature (-, +, +, +) we get
||2 using the signature (-, +, +, +) we get
- something  |
d  |
2 |
+ something  the others, the others, |
 ) is non-zero.
) is non-zero.
Achilles: I think I see your reasoning... we could get a null path if all the coordinates were zero, but that just can't happen in a path! So you mean to tell me that this is true even of light beams. Mmm.... So you're telling me that r must change along the world line of any particle or photon! But that begs a question, since r is always changing with  , does it increase or decrease with proper time
, does it increase or decrease with proper time  ?
?
Tortoise: To tell you the truth, I looked in the Green Book, and all it said was the "obviously" r must decrease with  , but I couldn't see anything obvious about that.
, but I couldn't see anything obvious about that.
Achilles: Well, let me try a thought experiment for a change. If you accept for the moment the claim that a particle fired toward the black hole will move so as to decrease r, then there is at least one direction for which dr/d < 0. Now imagine a particle being fired in any direction. Since dr/d
< 0. Now imagine a particle being fired in any direction. Since dr/d will be a continuous function of the angle in which the particle is fired, we conclude that it must always be negative.
will be a continuous function of the angle in which the particle is fired, we conclude that it must always be negative.
Tortoise: Nice try, my friend, but you are being too hasty (as usual). That argument can work against you: suppose that a particle fired away from the black hole will move (initially at least) so as to increase r, then your argument proves that r increases no matter what direction the particle is fired. Back to the drawing board.
Achilles: I see your point...
Tortoise (interrupting): Not only that. You might recall from Lecture 38 (or thereabouts) that the 4-velocity of as radially moving particle in free-fall is given by
| V* | = | d  |
, , 0, 0, E(1-2M/r)-1 | , |
so that the fourth coordinate, dt/d = E(1-2M/r)-1, is negative inside the horizon. Therefore, proper time moves in the opposite direction to coordinate time!
= E(1-2M/r)-1, is negative inside the horizon. Therefore, proper time moves in the opposite direction to coordinate time!
Achilles: Now I'm really confused. Does this mean that for r to decrease with coordinate time, it has to increase with proper time?
Tortoise: Yes. So you were (as usual) totally wrong in your reason for asserting that dr/d is negative for an inward falling particle.
is negative for an inward falling particle.
Achilles: OK. So now the burden of proof is on you! You have to explain what the hell is going on.
Tortoise: That's easy. You might dimly recall the equation
d  |
2 |
= | E2 - 1 + 2M/r |
d  |
2 |
= | 2M/r. |
Notice that this is constant and never zero, so that dr/d can never change sign during the trajectory of the particle, even as (in its comoving frame) it passes through the event horizon. Therefore, since r was initially decreasing with
can never change sign during the trajectory of the particle, even as (in its comoving frame) it passes through the event horizon. Therefore, since r was initially decreasing with  (outside, in "normal" space-time), it must continue to do so throughout its world line. In other words, photons that originate outside the horizon can never escape in their comoving frame. Now (and here's the catch), since there are some particles whose world-lines have the property that the arc-length parameter (proper time) decreases with increasing r, and since r is the unique coordinate in the stationary frame that plays the formal role of time, and further since, in any frame, all world lines must move in the same direction with respect to the local time coordinate (meaning r) as their parameter increases, it follows that all world lines must decrease r with increasing proper time. Ergo, Achilles, r must always decrease with increasing proper time
(outside, in "normal" space-time), it must continue to do so throughout its world line. In other words, photons that originate outside the horizon can never escape in their comoving frame. Now (and here's the catch), since there are some particles whose world-lines have the property that the arc-length parameter (proper time) decreases with increasing r, and since r is the unique coordinate in the stationary frame that plays the formal role of time, and further since, in any frame, all world lines must move in the same direction with respect to the local time coordinate (meaning r) as their parameter increases, it follows that all world lines must decrease r with increasing proper time. Ergo, Achilles, r must always decrease with increasing proper time  .
Of course, a consequence of all of this is that no light, communication, or any physical object, can escape from within the event horizon. They are all doomed to fall into the singularity.
.
Of course, a consequence of all of this is that no light, communication, or any physical object, can escape from within the event horizon. They are all doomed to fall into the singularity.
Achilles: But what about the stationary observer?
Tortoise: Interesting point...the quantity dt/d = E(1-2M/r)-1 is negative, meaning proper time goes in the opposite direction to coordinate time and also becomes large as it approaches the horizon, so it would seem to the stationary observer inside the event horizon that things do move out toward the horizon, but take infinitely long to get there. There is a catch, however, there can be no "stationary observer" according to the above analysis...
= E(1-2M/r)-1 is negative, meaning proper time goes in the opposite direction to coordinate time and also becomes large as it approaches the horizon, so it would seem to the stationary observer inside the event horizon that things do move out toward the horizon, but take infinitely long to get there. There is a catch, however, there can be no "stationary observer" according to the above analysis...
Achilles: Oh.
Exercise Set 15
1. Verify that the integral for the infalling particle diverges the case E = 1.
2. Mini-Black Holes How heavy is a black hole with event horizon of radius one meter? [Hint: Recall that the "M" corresponds to G total mass.]
total mass.]
3. Calculate the Riemann coordinates of curvature tensor Rabcd at the event horizon. r = 2M.
| Table of Contents | Back to Lecture 14: The Einstein Field Equations and Derivation of Newton's Law | On to Lecture 16: White Dwarfs, Neutron Stars, and Black Holes, by Gregory C. Levine |